Admin
مدير المنتدى


عدد المساهمات : 18996
التقييم : 35494
تاريخ التسجيل : 01/07/2009
الدولة : مصر
العمل : مدير منتدى هندسة الإنتاج والتصميم الميكانيكى
 |  موضوع: بحث بعنوان Structural Dynamic Modification موضوع: بحث بعنوان Structural Dynamic Modification  الإثنين 09 أغسطس 2021, 1:49 am الإثنين 09 أغسطس 2021, 1:49 am | |
| 
أخواني في الله
أحضرت لكم
بحث بعنوان
Structural Dynamic Modification
Dipartimento di Meccanica e Aeronautica, Universita` ‘‘La Sapienza’’, Rome, Italy
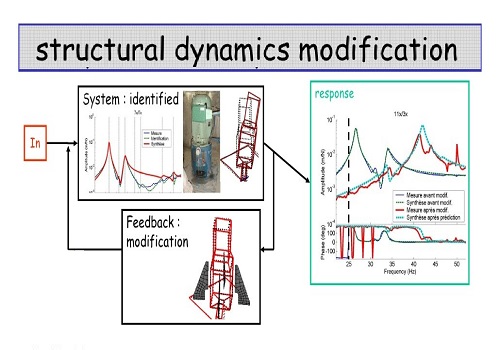
و المحتوى كما يلي :
Abstract. Vibration and acoustic requirements are becoming increasingly important in the design of mechanical structures, but they are not usually of primary
concern in the design process. So the need to vary the structural behaviour to
solve noise and vibration problems often occurs at the prototype stage, giving
rise to the so-called structural modification problem. In this paper, the direct
problem of determining the new response of a system, after some modifications
are introduced into the system, is analysed using two different databases: the
modal database and the frequency response function database. The limitations of
the modal database are discussed. Structural modifications that can be accounted
for are lumped masses, springs, dampers and dynamic absorbers.
Keywords. Structural dynamic modification; structural design; dynamic design; SDM using FRF.
Introduction
The problem of varying the dynamic behaviour of structures to reduce the vibration
response or control the noise radiated by vibrating surfaces is an exciting challenge. This is
particularly true if we refer to systems that were not originally designed to fulfil these
requirements. In fact, vibration and acoustics are not usually the primary concern of a
design project, although they increasingly become the focus of the problem once the
system has been built up. Unfortunately, the control of noise and vibration at this last stage,
often at project completion, is a very complex problem, either because the practical actions
are limited or because significant changes to the main features of the system are not
permitted. Moreover, when the considered situation is a running system, such as an engine,
a plant etc., the knowledge of the main dynamic parameters is poor and the system must be
supposed to be a black box, whose dynamics may be characterized by (experimental)
modal testing.
Structural modification (SM) is a procedure aimed at identifying the changes required in
a structural system to modify its dynamic behaviour (natural frequencies, structural modes, frequency response) in the desired direction. Enhancement of the structural or acoustic
response is one of the common goals of structural modification processes and can be
related to any of the following elements: the source, the transmission path or the noise
radiating component.
Two different problems are usually considered – the direct problem and the inverse
problem. The direct problem consists in determining the effect of already established
modifications. This is a verification problem aimed at establishing the efficiency of given
changes on the dynamic behaviour of the considered system. The inverse problem, more
complex, tries to identify, in the framework of a given set of possible modifications, the
most appropriate changes required to obtain the desired dynamic behaviour. Therefore this
is a typical design problem, consisting in specifying the required tools to obtain an
improvement of the structural or acoustic response.
Data describing the original vibrating structure, whose dynamic behaviour is modified,
can be obtained either from an experimental database or from theoretical analysis using a
finite element model of the system.
If a finite element database is available, the SM problem does not require an excessive
effort. But neither a finite element database nor the modal parameters of the system are
generally known, especially if a complex system is considered. This makes the problem
difficult to tackle. Therefore in recent years a great deal of attention has been devoted to
black box structures, dynamically identified through modal testing. The object of this rather
widespread effort was mainly the identification of spatial (elastic characteristics – i.e. mass
and stiffness matrices) and=or modal parameters, in order to acquire some knowledge of
the structure upon which to perform efficient modification. Since identification of spatial
parameters derived from an incomplete ‘‘mobility’’ model is a highly ill-conditioned problem, most of the work has been concentrated on modal parameters. Excluding particular
situations (high modal density, strongly coupled modes or high non-proportional damping),
modal parameters can be identified quite efficiently, at least in the frequency band tested,
and will produce useful albeit incomplete modal models. Once such a model is available,
many interesting structural modification algorithms can be developed and used with some
advantage.
Due probably to the degree of development of modal knowledge, most of the modification formulations apply to changes of modal characteristics, i.e. resonance shifts, Q-factor
reduction, optimal placement of nodal points etc., and the consequent synthesis is, in fact,
usually called ‘‘modal synthesis’’. This is not necessarily a limit although it does not
complete the wide field of applications that can be expected from structural modification.
Among these, some concern the need for reducing the vibration behaviour of particular
points of the structure in the frequency band of interest, or the possibility of narrowing the
rigid body natural frequencies of a suspended system to achieve the maximum isolation
efficiency (Johnson & Subhedar 1979).
Moreover, the incomplete modal model, identified from a continuous structure, severely
affects the results of structural modification. Wei et al (1987) showed that when using
modal parameters, the accuracy of the modified dynamic properties is largely affected by
the quality of the modal model derived from experimental data. Elliot & Mitchell (1987),
and Braun & Ram (1987), discussed the effect of modal truncation on structural and modal
modification. The former authors showed that the major role on the effectiveness of hardware modifications is played by a selected number of modes rather than by the quantity
of modes included in a database. Braun & Ram (1987) focused on the impossibility of
obtaining an exact solution for structural modification when using an incomplete set of eigenvectors. O’Callahan et al (1987) emphasized the effect of rigid body modes on
structural variations, and Wang et al (1987) discussed the effect of close modes and
incorrrect prediction of damping on sensitivity analysis.
For the above reasons, another modification approach is presented here (D’Ambrogio &
Sestieri 1987; Sestieri & D’ Ambrogio 1988, 1989). The method avoids a modal identification process and the pitfalls deriving from it. Moreover it may be used to handle useful
applications and permits optimal modifications to be accomplished with different dynamic
requirements.
The modifications considered are lumped modifications, which consist of any combination of lumped masses, stiffnesses (obtained by springs connecting two different points of
the structure or one point of the structure to an external fixed point) and damping.
Conclusions
The problem of structural modifications was presented in the framework of two different
approaches by using a modal database and an FRF database. The modal database presents
more limitations than the FRF approach, due to truncation effects in the former case.
When dealing with structural modifications, either a direct problem or an optimization
one can be considered. The first establishes the dynamic behaviour of the modified structure
once a set of changes has been established. In this context both the modal and the FRF
approaches are successful, although with the limitations examined for the modal database
the inverse or optimization problem tries to find the optimum set of modifications that
accomplishes the required dynamic behaviour of the system. The use of the FRF database
is particularly appropriate to give a satisfactory solution of the problem, while this is not
definitely the case with the modal approach.
كلمة سر فك الضغط : books-world.net
The Unzip Password : books-world.net
أتمنى أن تستفيدوا من محتوى الموضوع وأن ينال إعجابكم
رابط من موقع عالم الكتب لتنزيل بحث بعنوان Structural Dynamic Modification
رابط مباشر لتنزيل بحث بعنوان Structural Dynamic Modification 
|
|







